direct sums, tensor products, homomorphisms

The first definition in the previous subsection is important because one may easily recognize that the following type of lemma should hold.

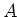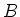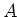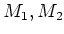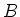LEMMA 1.1   Let $A$ be a commutative ring. Let $B$ be a commutative $A$ -algebra. Let $M_1,M_2$ be $B$ -modules with connections

$$\nabla_j: M_j\to \Omega^1_{B/A} \otimes_B M_j \qquad (j=1,2).$$

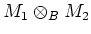Then we may define a connection on the direct sum $M_1\otimes M_2$ , on the tensor product $M_1\otimes_B M_2$ , and on the module 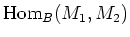$\operatorname{Hom}_B(M_1, M_2)$ . Namely,

$$\nabla(m_1,m_2)=(\nabla_1(m_1), \nabla_2 (m_2))$$

$$\nabla(m_1\otimes m_2)=\nabla_1(m_1)\otimes m_2 + m_1\otimes \nabla_2 (m_2)$$

$$\nabla(\phi)(s)=\nabla_2(\phi(s))-(\operatorname{id}_{\Omega^1}\otimes \phi)(\nabla_1(s))$$

PROOF.. Easy.

$\qedsymbol$