-
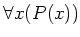 は 「どのような
は 「どのような 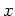 にたいしても、
にたいしても、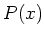 が成り立つ」
という意味である。
が成り立つ」
という意味である。
-
 は 「少なくとも一つの
は 「少なくとも一つの 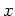 にたいして、
にたいして、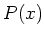 が成り立つ」
という意味である。
が成り立つ」
という意味である。
原理的には、![]() ,
, ![]() および前回の論理記号と、
集合論の幾つかの記号を組み合わせることにより、数学の
すべての言葉を記号列に翻訳できる。
および前回の論理記号と、
集合論の幾つかの記号を組み合わせることにより、数学の
すべての言葉を記号列に翻訳できる。
例えばつぎの文は、「数学的帰納法」を表現したものである。
また、つぎの文章は、「
![]() は位相空間である」
ということ(の定義)を述べたものである。
は位相空間である」
ということ(の定義)を述べたものである。
数学における論理は、 これらの記号列に関する「単純な計算」である。 つまり、「論理を追う」とは、これらの単純な計算を 間違いなく素早く行なうことである。それは、 かけ算の九九と同じように、 練習をすればほとんど誰でもできる。 ただし、つぎのような練習が必要である。
さしあたって今回は、つぎのことに注意しよう。
登場人物(![]() や
や ![]() などの変数)の出てくる順番が
大事である。
などの変数)の出てくる順番が
大事である。
例えば、
![]() グー、チョキ、パー
グー、チョキ、パー![]() , にたいして、
, にたいして、
![]() を、「
を、「![]() は
は ![]() より強い」というふうに定義すると。
より強い」というふうに定義すると。
は真の命題だが、
は偽の命題である。
は真の命題だが、
は偽の命題である ようなものを挙げ、どうしてそうなのか解説を書きなさい。
&dotfill#dotfill;
(補遺)
はきちんと書くと
という意味、
はきちんと書くと
という意味の省略形である。