Commutative algebra

Yoshifumi Tsuchimoto

LEMMA 05.1   Let

$$0 \to L \to M \to N\to 0$$

be an exact sequence of $A$ -modules. Then we have

$$l(L)+l(N)=l(M).$$

DEFINITION 05.2   Let $A=\oplus_{i=0}^\infty A_i$ be a graded algebra. We assume

1. $l_{A_0}(A_0)<\infty$ (Length of $A_0$ as an $A_0$ module is finite.)
2. $A$ is generated by homogeneous elements $x_1,x_2,\dots, x_r$ where $\deg(x_i)=d_i$ .

Then for any graded finite $A$ -module $M$ , We define its Hilbert series as

$$\varphi_M(t)=\sum_{j=0}^\infty l_{A_0}(M_j) t^j$$

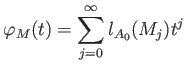
PROPOSITION 05.3   Under the assumption of the definition above, The Hilbert series $\varphi_M$ is a rational function on $t$ . More precisely, we have

$$\prod_{j=1}^r (1-t^{d_j}) \varphi_M(t) \in \mathbb{Q}[t]$$

PROPOSITION 05.4   If a graded algebra is generated by $x_1,x_2,\dots, x_r$ of degree $1$ over a ring $A_0$ with $l_{A_0}(A_0)<\infty$ , there exists a polynomial $p_M$ such that

$$l(M_k)=p_M(k) \quad (\forall k »0).$$

We call $p_M$ the Hilbert polynomial of $M$ .

COROLLARY 05.5   Let $(A,\mathfrak{m})$ be a Noetherian local ring. Let $I$ be an ideal of definition (That means, there exists $n_0$ such that $\mathfrak{m} \supset I\supset \mathfrak{m}^{n_0}$ holds.) We put $\chi^I_M(j)=l(M/I^j)$ . Then there exists a polynomial $p$ such that $p(j)=\chi^I_M(j)$ holds for $j»0$ .

DEFINITION 05.6   Under the hypothesis of the Corollary above, we define the Samuel function of $M$ as $\chi_M^{\mathfrak{m}}(\bullet)$ .

THEOREM 05.7 (Nakayama's lemma, or NAK)   Let $A$ be a commutative ring. Let $M$ be an $A$ -module. We assume that $M$ is finitely generated (as a module) over $A$ . That means, there exists a finite set of elements $\{m_i\}_{i=1}^t$ such that

$$M=\sum_{i=1}^t A m_i$$

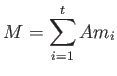
holds. If an ideal $I$ of $A$ satisfies

$$I M=M \quad ($$that is, $$M/I M=0),$$

then there exists an element $c\in I$ such that

$$c m= m \qquad (\forall m \in M)$$

holds. If furthermore $I$ is contained in $\cap_{\mathfrak{m}\in \operatorname{Spm}(A)} \mathfrak{m}$ (the Jacobson radical of $A$ ), then we have $M=0$ .

PROOF.. Since $I M=M$ , there exists elements $b_{i l}\in I$ such that

$$a_i= \sum_{l=1}^t b_{i l} a_l \qquad (1\leq i \leq t )$$

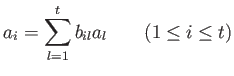
holds. In a matrix notation, this may be rewritten as

$$v=B v$$

with $v=^t (m_1,\dots,m_n)$ , $B=(b_{i j})\in M_t (I)$ . Using the unit matrix $1_t\in M_t(A)$ one may also write :

$$(1_t -B) v=0.$$

Now let $R$ be the adjugate matrix of $1_t -B$ . In other words, it is a matrix which satisfies

$$R(1_t-B)=(1_t-B)R=(\det(1_t-B)) 1_t.$$

Then we have

$$\det(1_t -B)\cdot v = R (1_t -B) v=0.$$

On the other hand, since $1_t-B=1_t$ modulo $I$ , we have $\det(1_t-B)=1-c$ for some $c\in I$ . This $c$ clearly satisfies

$$v=c v.$$

$\qedsymbol$